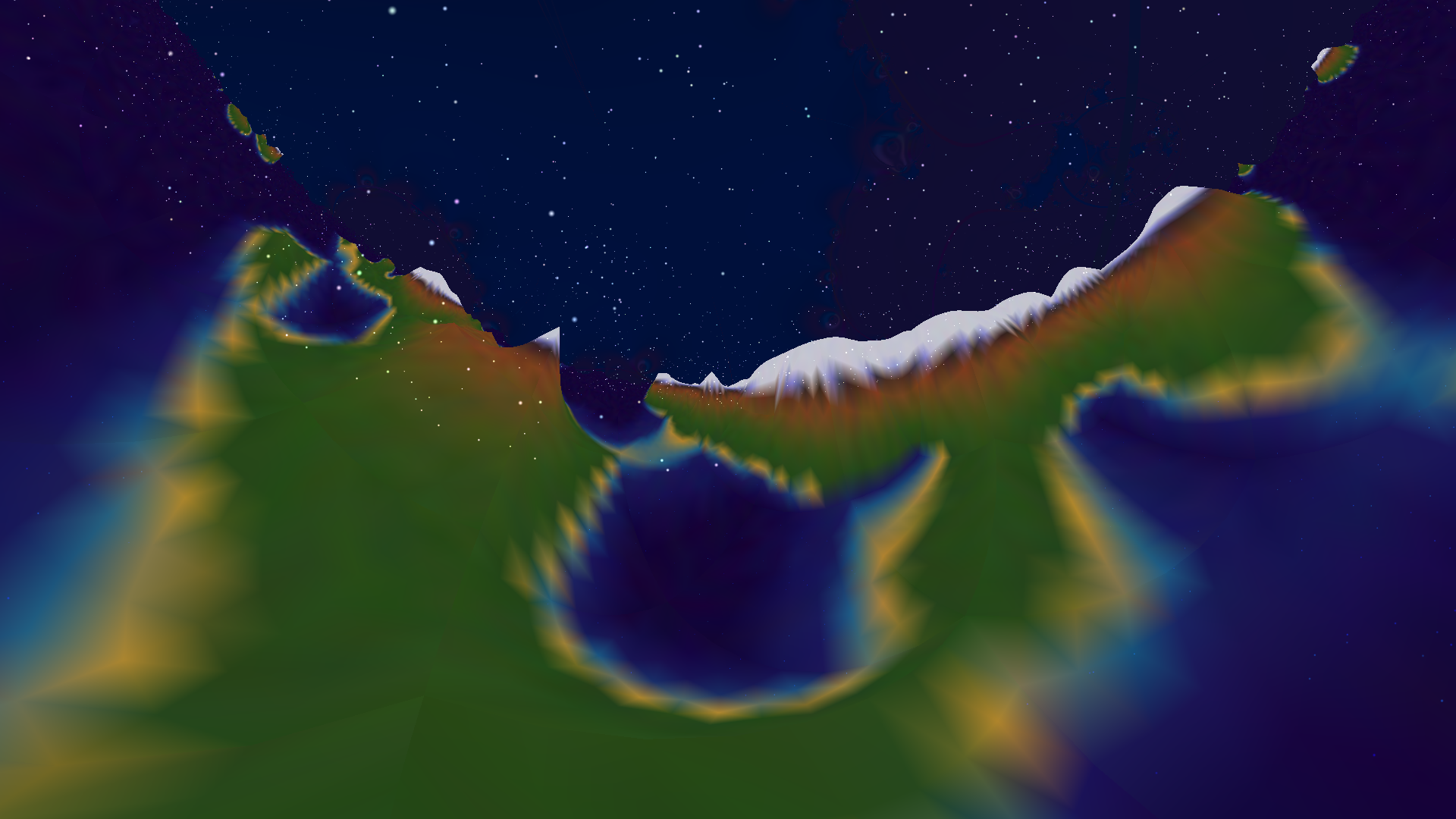
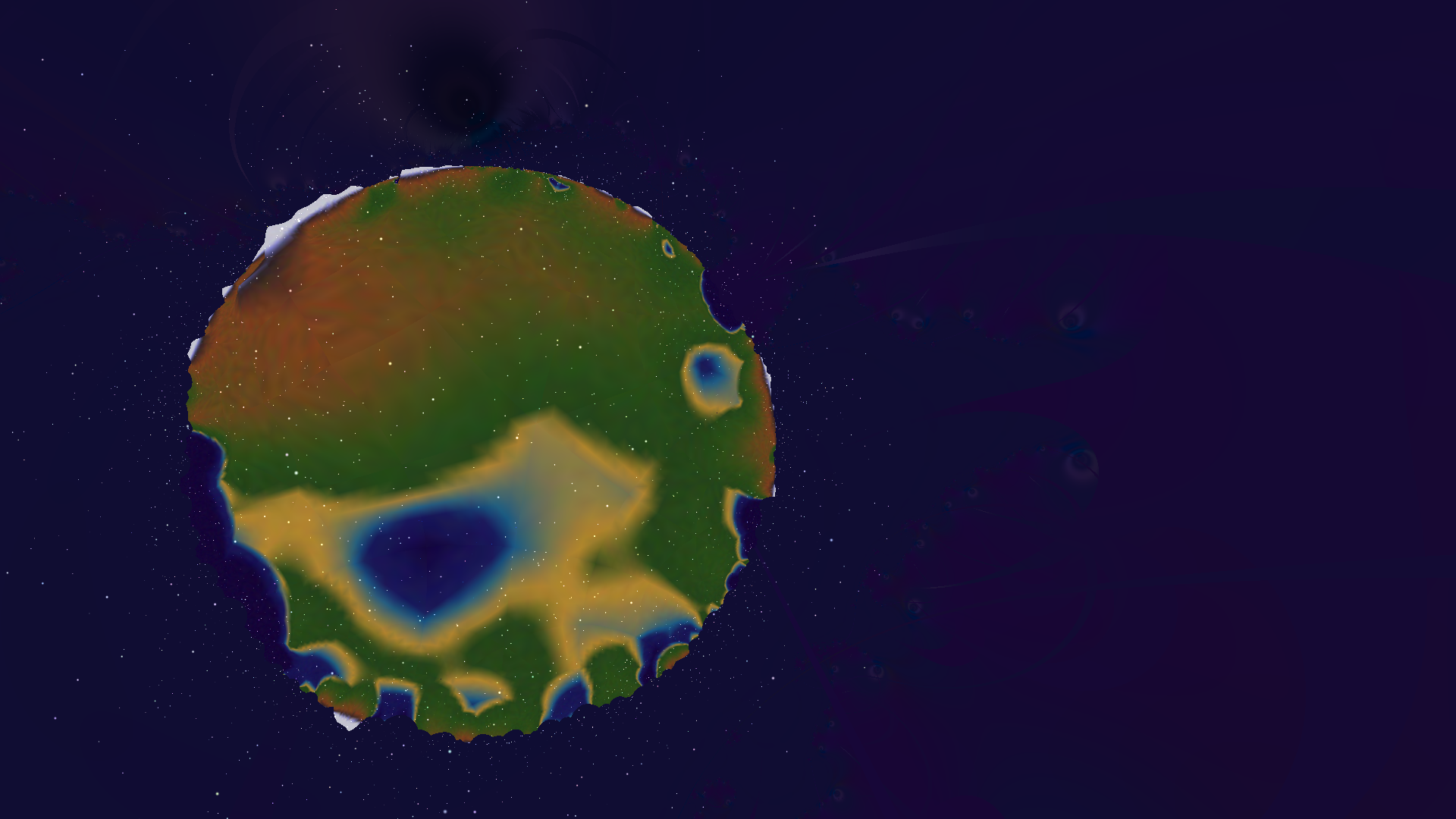
Low-poly procedural terrain generation based on an Order-4 Hexagonal Tiling.
Contains a simple treasure hunt game to incentivize exploration!
Terrain features are based on elevation relative to a reference plane,
randomized per-tile with deviations according to a preset type, from gentle to extreme.
Parameterized
Triangle Group
viewer.
Hyperbolic, Parabolic (Euclidean), and Spherical tessellations can all be represented.
Fractal
patterns can be generated by iterative functions in the complex plane.
Such images are usually interpreted as displaying structure at arbitrary scales, unfolded by magnification.
The complex plane can be mapped to the Riemann Sphere,
and rendered as the "horizon" of hyperbolic space, an infinite distance from the camera.
Under this interpretation, fractal structure in its natural habitat on the horizon is unfolded by
motions through 3D hyperbolic space, with the apparent magnification as an effect of perspective.
Visualize binary trees in 3D hyperbolic geometry, with random or customized growth parameters.
The recursive nature of the branching creates a canopy of fractal forms.
Exponential growth inevitably outpaces the amount of space available to a Euclidean tree.
A hyperbolic tree with the right configuration however, can continue to grow unhindered by a lack of room.
As branches extend, space itself diverges to accommodate the next layer of new growth.
Clouds of aether illuminate an otherwise pitch-black void of hyperbolic space.
Symmetries are generated by a
Kleinian Group
of four generators, with varying parameters.
